The solution to the “Where Wolf?”
puzzle is now available:
#5: Where Wolf?
A truth-tellers and liars puzzle.
Published: 12/06/2015
Difficulty: **
Maths knowledge required: None
There Wolf!
Leave a reply
Monthly Archives: June 2015
The number that just won’t go away…
The 82000 sequence is still generating interest.
Here’s a great Numberphile video about the sequence from mathematician James Grime:
[youtube http://www.youtube.com/watch?v=LNS1fabDkeA]
The sequence has also made it into the Online Encyclopaedia of Integer Sequences, as A258107!
I have also found an early mention of the special properties of 82000, in this exchange on a French puzzle forum, from October 2008. Continue reading
Puzzle #5: Where Wolf?
So I promised a really hard puzzle for #5… Well, this is not that one, sorry. Next time. This is a late substitute. A good one though.
A maths joke
A mathematician walks into a bar. The punchline is left as an exercise for the reader. #mathsjoke
— Thomas Oléron Evans (@Mathistopheles) March 17, 2015
Probabilities for the French Open final
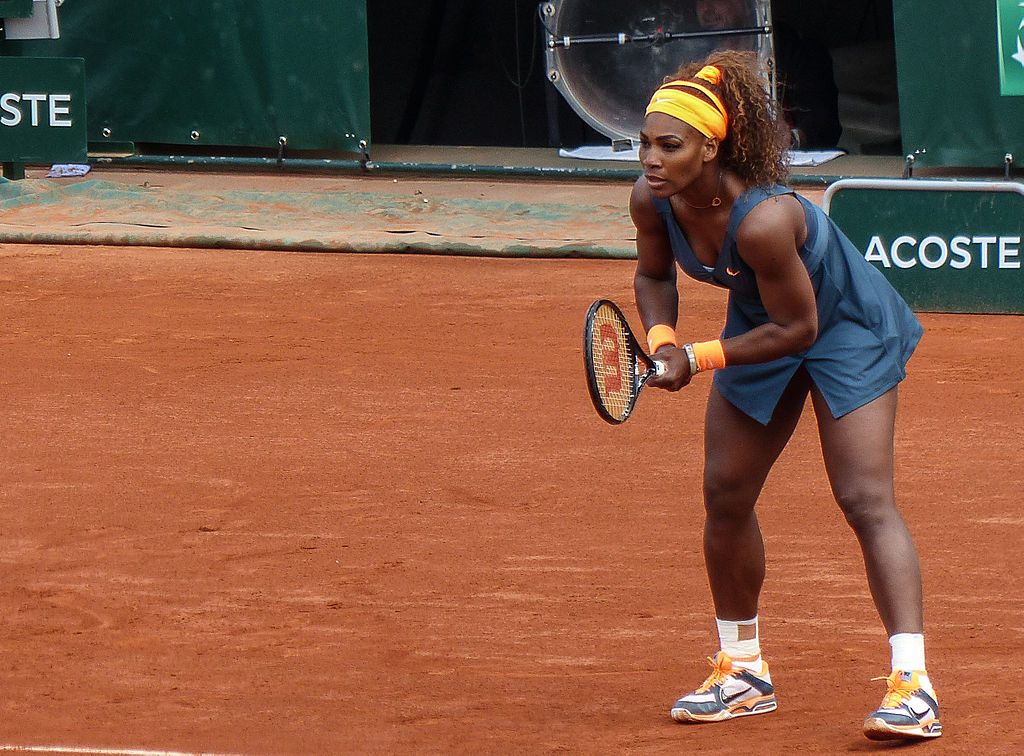
Here’s a bit of very simplistic probability calculation on the outcome of the French Open final, related to my article on the value of a set in tennis. Continue reading
Tennis: How much is a set worth?
.
With the men’s final of Roland Garros about to start, I have written a pretty simple piece about how much a set is worth in a tennis match (in probability terms). I have tried to keep the style accessible, as with the Pointless maths articles (although I probably simplified a little too much with those).
Click here to read the article.
The photo is from Yann Caradec via Wikimedia Commons, published Creative Commons: HERE.
The solution to the algebra puzzle…
The solution to Puzzle #4 is now up. Either follow the links from the puzzle itself HERE, or, if you have seen the question already, click HERE to go straight to the solution.
As I mentioned previously, I think this is a good puzzle for mathematics learners at GCSE level or for those setting out on A-level courses, both for checking up on basic algebraic understanding and for illustrating some specific problem-solving skills. I have summarised the key learning points that I think the puzzle raises on the solution page.
New Puzzle
I have put a new puzzle up. Nothing too tough this time. A classic algebra puzzle, which I have found to be pretty good as a test of how well GCSE/A-level students understand some basic points of algebra, while illustrating some important problem solving skills.
#4: Hidden depths…
A classic algebra puzzle.
Published: 02/06/2015
Difficulty: *
Maths knowledge required: GCSE algebra [i.e. 14-16 year olds]. Although KS3 would do…
Click HERE to access the puzzle.
The solution will go up in a day or two.
Fractal Follow-up
There have been a few nice follow-ups on my fractals based on iterating the factorial function:
- Professor Richard Green posted his own commentary on my images.
- Mathematician, geek, wordsmith, singer, problem-solver and uncle Steve Kass made some Gamma function fractals of his own.
- Twitter user @mathinpython has produced a great step-by-step guide to creating factorial fractals in Python.
Steve Kass also directed me to this page, in which academic multitasker in computer science, neuroscience, philosophy and future studies Anders Sandberg presents some lovely alternative Gamma function fractals (posted in February). Intriguingly, the author has also co-written an academic paper on the science of tie-knots (apparently inspired by a costume from The Matrix Reloaded), which identifies “266,682 distinct tie-knots that seem tie-able with a normal neck-tie”. Surely worth a read.
In other news, I recently passed my PhD viva, which is probably for the best. Among other things, this means that my putative article on “How to fail your PhD” will have to be put on ice indefinitely.